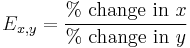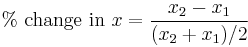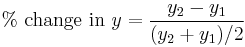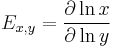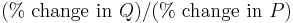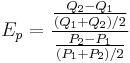Arc elasticity
Arc elasticity is the elasticity of one variable with respect to another between two given points.
Contents |
Formula
The y arc elasticity of x is defined as:
where the percentage change is calculated relative to the midpoint
The midpoint arc elasticity formula was advocated by R. G. D. Allen due to the following properties: (1) it is symmetric with respect to the two prices and two quantities, (2) it is independent of the units of measurement, and (3) it yields a value of unity if the total revenues at two points are equal.[1]
Arc elasticity is used when there is not a general function for the relationship of two variables. Therefore, point elasticity may be seen as an estimator of elasticity; this is because point elasticity may be ascertained whenever a function is defined.
For comparison, the y point elasticity of x is given by:
Application in economics
The P arc elasticity of Q is calculated as
The percentage is calculated differently from the normal manner of percent change. This percent change uses the average (or midpoint) of the points, in lieu of the original point as the base.
Example
Suppose that you know of two points on a demand curve 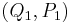 and
and 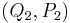 . (Nothing else might be known about the demand curve.) Then you obtain the arc elasticity (a measure of the price elasticity of demand and an estimate of the elasticity of a differentiable curve at a single point) using the formula
. (Nothing else might be known about the demand curve.) Then you obtain the arc elasticity (a measure of the price elasticity of demand and an estimate of the elasticity of a differentiable curve at a single point) using the formula
Suppose we measure the demand for hot dogs at a football game. Let's say that after halftime we lower the price, and quantity demanded changes from 80 units to 120 units. The percent change, measured against the average, would be (120-80)/((120+80)/2))=40%.
Normally, a percent change is measured against the initial value. In this case, this gives (12-8)/8= 50%. The percent change for the opposite trend, 120 units to 80 units, would be -33.3%. The midpoint formula has the benefit that a movement from A to B is the same as a movement from B to A in absolute value. (In this case, it would be -40%.)
Suppose that the change in the price of hot dogs was from $3 to $1. The percent change in price measured against the midpoint would be -100%, so the price elasticity of demand is (40%/-100%) or -40%. It is common to use the absolute value of price elasticity, since for a normal (decreasing) demand curve they are always negative. Thus the demand of the football fans for hot dogs has 40% elasticity, and is therefore inelastic.
See also
References
- ^ R. G. D. Allen, 1933, The concept of arc elasticity of demand. Review of Economic Studies, 1(3), pp.226-229